and the distribution of digital products.
Unveiling Path Signatures: A Key to Geometric Insights in Data
:::info Authors:
(1) Guillaume Staerman, INRIA, CEA, Univ. Paris-Saclay, France;
(2) Marta Campi, CERIAH, Institut de l’Audition, Institut Pasteur, France;
(3) Gareth W. Peters, Department of Statistics & Applied Probability, University of California Santa Barbara, USA.
:::
Table of Links2.1. Functional Isolation Forest
3. Signature Isolation Forest Method
4.1. Parameters Sensitivity Analysis
4.2. Advantages of (K-)SIF over FIF
4.3. Real-data Anomaly Detection Benchmark
5. Discussion & Conclusion, Impact Statements, and References
\ Appendix
A. Additional Information About the Signature
C. Additional Numerical Experiments
2.2. The Signature MethodThe signature of a path is a sequence of iterated integrals that captures important information about the path’s geometric and topological features (Lyons et al., 2007; Fermanian, 2021).
\
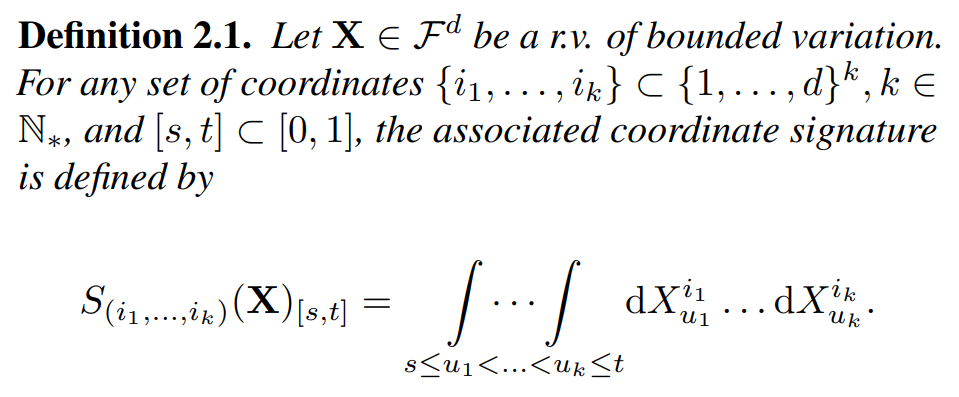
\ Furthermore, the signature of X is defined as the infinite collection of coordinate signature
\
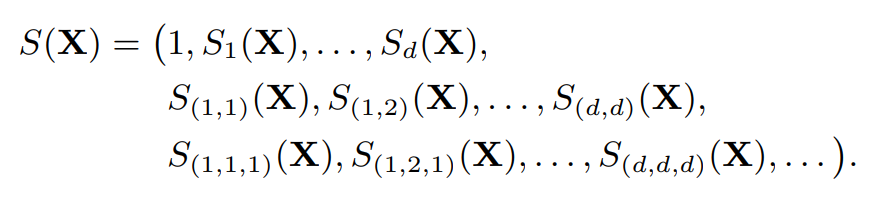
\

\
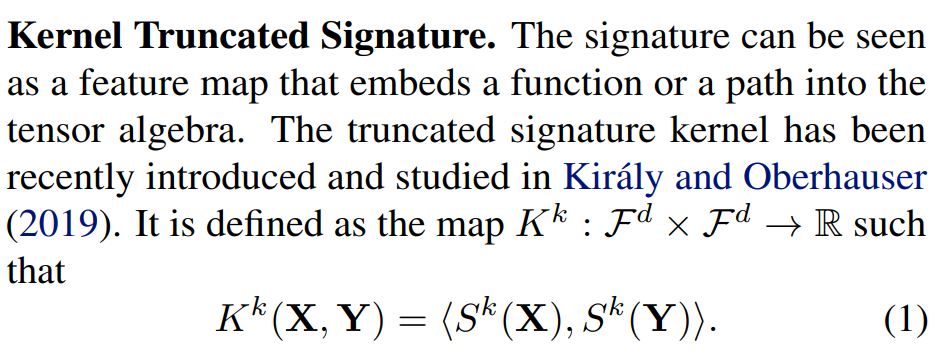
\ One may refer to Lee and Oberhauser (2023) for an account of the untruncated kernel signature. See also Section A in the Appendix for further details about the signature and its properties.
\
:::info This paper is available on arxiv under CC BY 4.0 DEED license.
:::
\
- Home
- About Us
- Write For Us / Submit Content
- Advertising And Affiliates
- Feeds And Syndication
- Contact Us
- Login
- Privacy
All Rights Reserved. Copyright , Central Coast Communications, Inc.