and the distribution of digital products.
Bernstein’s Concentration Inequality In Equivalence Testing
:::info Authors:
(1) Diptarka Chakraborty, National University of Singapore, Singapore
(2) Sourav Chakraborty, Indian Statistical Institute, Kolkata;
(3) Gunjan Kumar, National University of Singapore, Singapore;
(4) Kuldeep S. Meel, University of Toronto, Toronto.
:::
Table of Links4 An Efficient One-Round Adaptive Algorithm and 4.1 High-Level Overview
6 Acknowledgements and References
B An O(log log n)-query fully adaptive algorithm
A MISSING PROOFS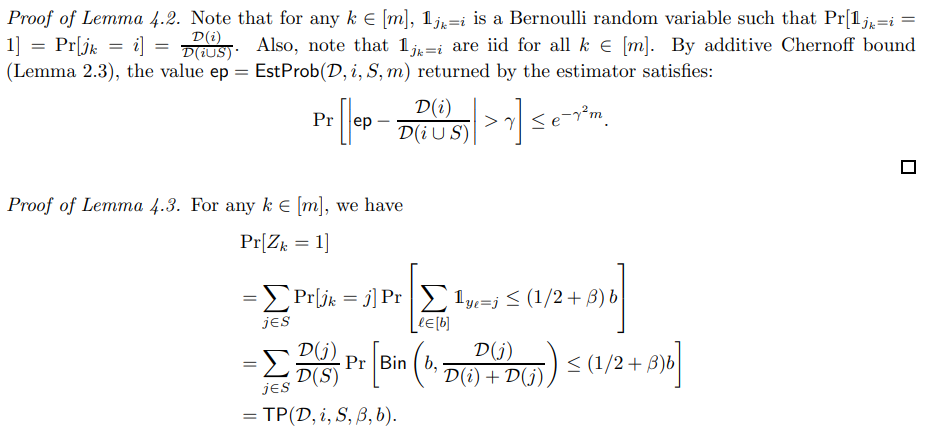
\ Therefore, by additive Chernoff bound (Lemma 2.3), the value et = EstTail(D, i, S, β, b, m) returned by the algorithm satisfies
\
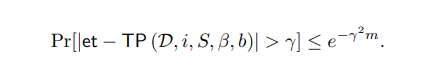
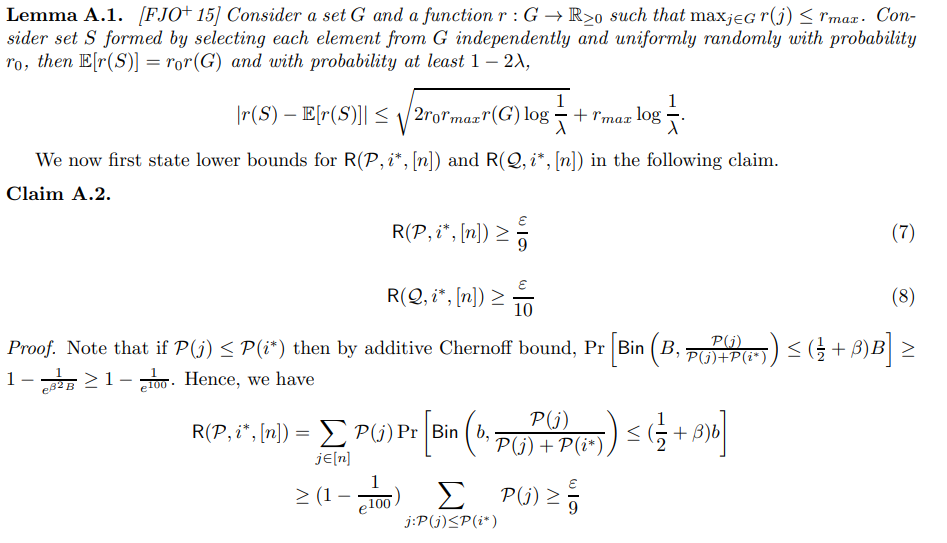
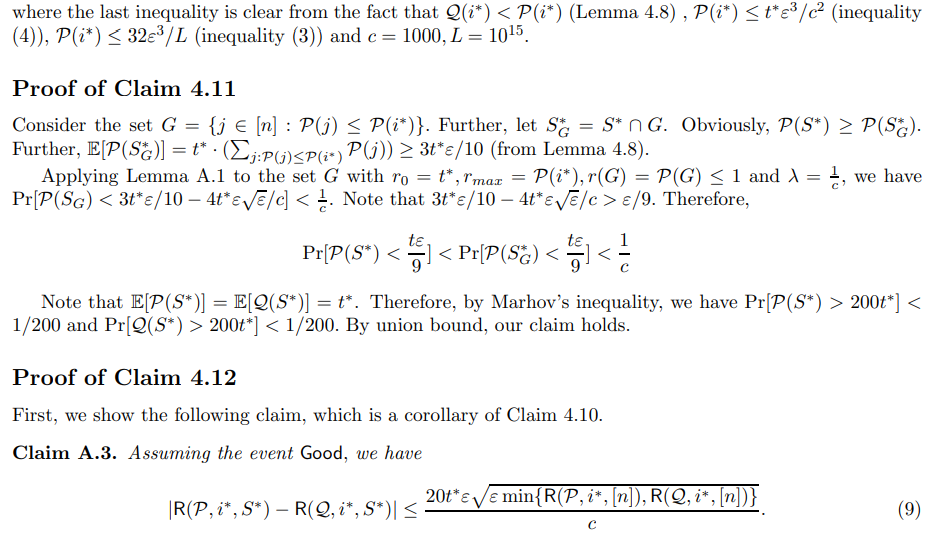
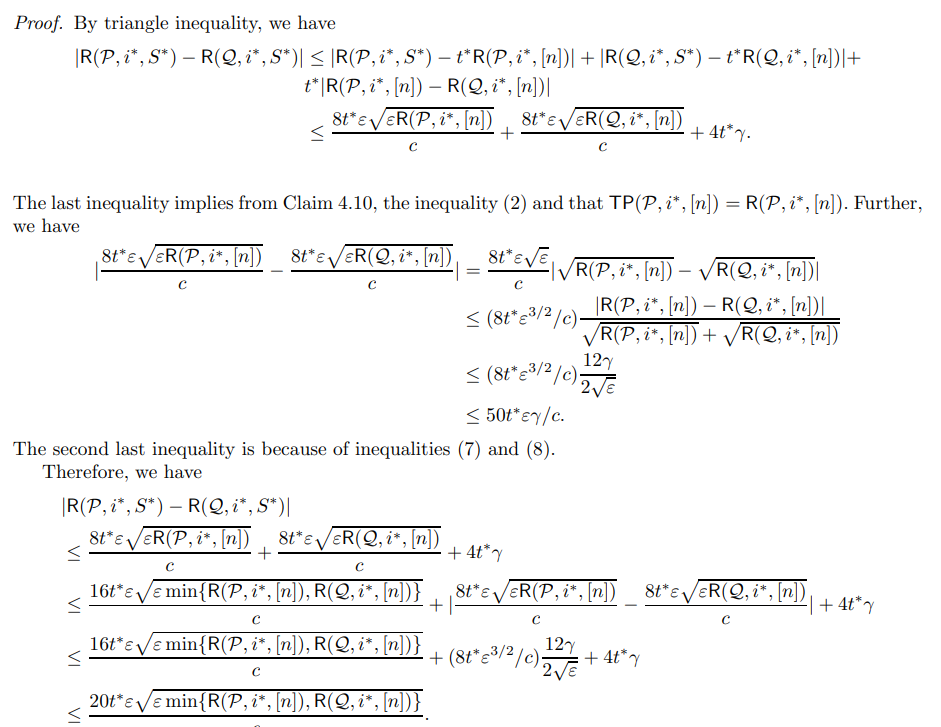
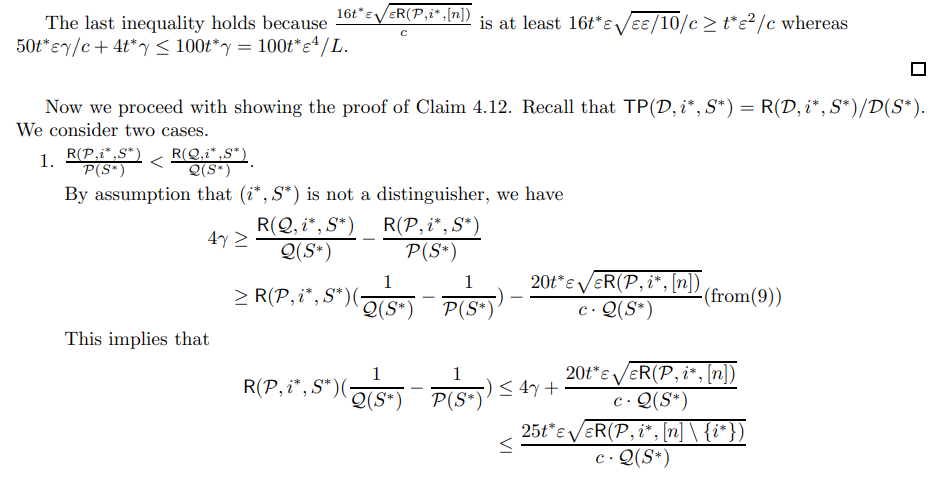
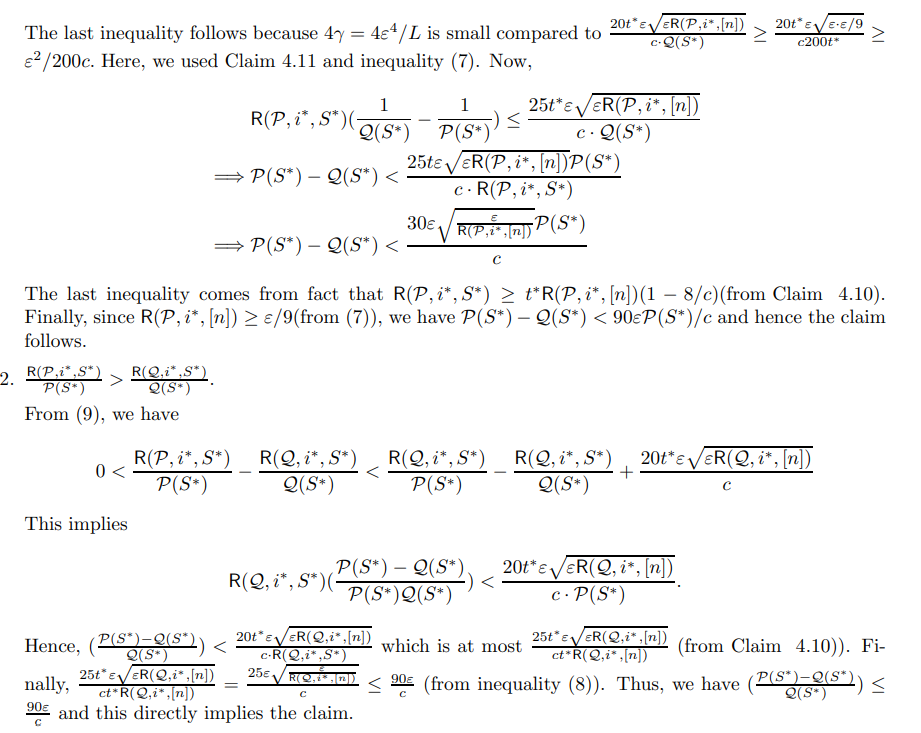
\ To prove Claims 4.10, 4.11 and 4.12, we will use the following concentration inequality that directly follows from Bernstein’s concentration inequality.
\
\
\
\
\
\
\
:::info This paper is available on arxiv under CC BY-NC-SA 4.0 DEED license.
:::
\